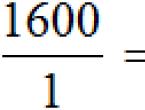Proportions. Solving problems using proportions How to make a proportion

Basic properties of proportions

Reversal of proportion. If a : b = c : d, That b : a = d : c
Multiplying the terms of a proportion crosswise. If a : b = c : d, That ad = bc.
Rearrangement of middle and extreme terms. If a : b = c : d, That

a : c = b : d(rearrangement of the middle terms of the proportion), d : b = c : a(rearrangement of the extreme terms of the proportion).

Increasing and decreasing proportions. If a : b = c : d, That

(a + b) : b = (c + d) : d (increase in proportion), (a – b) : b = (c – d) : d (decrease in proportion).

Making proportions by adding and subtracting. If a : b = c : d, That

(a + With) : (b + d) = a : b = c : d(composing proportions by addition), (a – With) : (b – d) = a : b = c : d(composing proportions by subtraction).

Composite (continuous) proportions

Historical reference

Literature

van der Waerden, B. L. Awakening Science. Mathematics of Ancient Egypt, Babylon and Greece. - per. from Dutch I. N. Veselovsky- M.: GIFML, 1959

How to solve a problem using proportion

Let's look at a simple example. Three groups need to be paid a stipend of 1,600 rubles each. There are 20 students in the first group. This means that the first group will be paid 1600 × 20, that is, 32 thousand rubles.

There are 17 people in the second group. This means that the second group will be paid 1600 × 17, that is, 27,200 thousand rubles.

Well, we’ll pay a stipend to the third group. There are 15 people in it. You need to spend 1600 × 15 on them, that is, 24 thousand rubles.

As a result, we have the following solution:

For such problems, the solution can be written using a proportion.

Proportion by definition is the equality of two ratios. For example, equality is proportion. This proportion can be read as follows:

a this applies to b, How c applies d

Similarly, you can correlate the scholarship and students, so that each gets 1,600 rubles.

So, let’s write down the first ratio, namely the ratio of one thousand six hundred rubles per person:

We found out that to pay 20 students 1,600 rubles each, we will need 32 thousand rubles. So the second ratio will be the ratio of thirty-two thousand to twenty students:

Now we connect the resulting relations with an equal sign:

We got the proportion. It can be read as follows:

One thousand six hundred rubles relate to one student as thirty-two thousand rubles relate to twenty students.

That is, 1600 rubles each. If you divide on both sides of the equation , then we will find that one student, like twenty students, will receive 1,600 rubles.

Now imagine that the amount of money needed to pay scholarships to twenty students was unknown. Let's say if the question was like this: V There are 20 students in the group and each needs to pay 1600 rubles. How many rubles are required to pay the scholarship?

In this case the proportion would take the form. That is, the amount of money needed to pay the scholarship has become an unknown member of the proportion. This proportion can be read as follows:

One thousand six hundred rubles relates to one student as unknown number of rubles refers to twenty students

Now let's use the basic property of proportion. It states that the product of the extreme terms of a proportion is equal to the product of the middle terms:

Multiplying the terms of the proportion “crosswise”, we get the equality 1600 × 20 = 1 × x. Having calculated both sides of the equality, we get 32000 = x or x= 32000 . In other words, we will find the value of the unknown quantity that we were looking for.

Similarly, it was possible to determine the total amount for the remaining number of students - for 17 and 15. These proportions looked like and. Using the basic property of proportion, you can find the value x

Problem 2. The bus covered a distance of 100 km in 2 hours. How long will it take the bus to travel 300 km if it travels at the same speed?

You can first determine the distance the bus travels in one hour. Then determine how many times this distance is contained in 300 kilometers:

100: 2 = 50 km for every hour of travel

300 km: 50 = 6 hours

Or you can make the proportion “one hundred kilometers are to one hour as three hundred kilometers are to an unknown number of hours”:

Ratio of like quantities

If the extreme or middle terms of the proportion are swapped, the proportion will not be violated.

Yes, in proportion you can swap the extreme members. Then you get the proportion .

The proportion will also not be violated if it is turned upside down, that is, inverse ratios are used in both parts.

Let's reverse the proportion . Then we get the proportion . The relationship is not broken. The ratio between students is equal to the ratio between the amounts of money intended for these students. This proportion is often drawn up in school when tables are compiled to solve a problem.

This writing method is very convenient because it allows you to translate the problem statement into a more understandable form. Let's solve a problem in which we needed to determine how many rubles are needed to pay scholarships to twenty students.

Let us write the problem conditions as follows:

Let's create a table based on this condition:

Let's make a proportion using the table data:

Using the basic property of proportion, we obtain a linear equation and find its root:

Initially, we were dealing with proportion , which is made up of ratios of quantities of different natures. The numerators of the ratios contained the amounts of money, and the denominators included the number of students:

By swapping the extreme members, we get the proportion . This proportion is made up of ratios of quantities of the same nature. The first relation contains the number of students, and the second – the amount of money:

If a relation is composed of quantities of the same nature, then we will call it ratio of quantities of the same name. For example, the relationship between fruits, money, physical quantities, phenomena, actions.

A ratio can be composed both from quantities of the same name and from quantities of different natures. Examples of the latter are the ratio of distance to time, the ratio of the cost of a product to its quantity, and the ratio of the total amount of scholarships to the number of students.

Example 2. Pine and birch trees are planted in the school garden, with 2 birches for every pine tree. How many pine trees were planted in the garden if 240 birch trees were planted?

Let's determine how many pine trees were planted in the garden. To do this, let's create a proportion. The condition says that for every pine tree there are 2 birches. Let's write a relation showing that there are two birches for one pine tree:

Now let's write a second relation showing that x pine trees account for 240 birches

Let's connect these relations with an equal sign and get the following proportion:

“Two birches treat one pine tree like this,
how 240 birches relate to x pine trees"

Using the basic property of proportion, we find the value x

Or the proportion can be made by first writing down the condition, as in the previous example:

You will get the same proportion, but this time it will be made up of ratios of quantities of the same name:

This means that 120 pine trees were planted in the garden.

Example 3. From 225 kg of ore, 34.2 kg of copper were obtained. What is the percentage of copper in the ore?

You can divide 34.2 by 225 and express the result as a percentage:

Or make a proportion of 225 kilograms of ore as 100%, as 34.2 kg of copper are at an unknown number of percent:

Or create a proportion in which the ratios are made up of quantities of the same name:

Direct proportionality problems

Understanding the relationships of quantities of the same name leads to an understanding of solving problems of direct and inverse proportionality. Let's start with direct proportionality problems.

First, let's remember what direct proportionality is. This is a relationship between two quantities in which an increase in one of them entails an increase in the other by the same amount.

If a bus covered a distance of 50 km in 1 hour, then to cover a distance of 100 km (at the same speed) the bus would take 2 hours. As the distance increased, the travel time increased by the same amount. How to show this using proportion?

One of the purposes of the ratio is to show how many times the first quantity is greater than the second. This means that using proportions we can show that distance and time have doubled. To do this, we use the ratio of quantities of the same name.

Let us show that the distance has doubled:

Similarly, we will show that the time has increased by the same amount

“100 kilometers are to 50 kilometers as 2 hours are to 1 hour”

If we divide on both sides of the equation, we will find that the distance and time have been increased by the same number of times.

2 = 2

Problem 2. In 3 hours, 27 tons of wheat flour were ground at the mill. How many tons of wheat flour can be milled in 9 hours if the work rate does not change?

Solution

The operating time of the mill and the mass of ground flour are directly proportional quantities. By increasing the operating time several times, the amount of ground flour will increase by the same amount. Let's show this using proportion.

In the problem, 3 hours are given. These 3 hours increased to 9 hours. Let us write the ratio of 9 hours to 3 hours. This ratio will show how many times the mill’s operating time has increased:

Now let's write down the second relation. It will be an attitude x tons of wheat flour to 27 tons. This ratio will show that the amount of milled flour has increased by the same amount as the operating time of the mill

Let's connect these relations with an equal sign and get proportion.

Let's use the basic property of proportion and find x

This means that in 9 hours you can grind 81 tons of wheat flour.

In general, if you take two directly proportional quantities and increase them by the same number of times, then the ratio of the new value to the old value of the first quantity will be equal to the ratio of the new value to the old value of the second quantity.

So in the previous problem, the old values were 3 h and 27 t. These values were increased by the same number of times (three times). The new values are 9 hours and 81 hours. Then the ratio of the new value of the mill operating time to the old value is equal to the ratio of the new value of the mass of ground flour to the old value

If we divide on both sides of the equation, we will find that the operating time of the mill and the amount of milled flour have increased by the same number of times:

3 = 3

The proportion that is added to direct proportionality problems can be described using the expression:

Where later it became equal to 81.

Problem 2. For 8 cows in winter, the milkmaid daily prepares 80 kg of hay, 96 kg of root crops, 120 kg of silage and 12 kg of concentrates. Determine the daily consumption of this feed for 18 cows.

Solution

The number of cows and the weight of each feed are directly proportional. When the number of cows increases several times, the weight of each feed will increase by the same amount.

Let's make several proportions that calculate the mass of each feed for 18 cows.

Let's start with the hay. Every day 80 kg of it are prepared for 8 cows. Then 18 cows will be prepared x kg of hay.

Let's write down a ratio showing how many times the number of cows has increased:

Now let’s write down the ratio showing how many times the mass of hay has increased:

Let's connect these relations with an equal sign and get the proportion:

From here we find x

This means that for 18 cows you need to prepare 180 kg of hay. Similarly, we determine the mass of root crops, silage and concentrates.

For 8 cows, 96 kg of root crops are harvested daily. Then 18 cows will be prepared x kg of root vegetables. Let's make a proportion from the ratios and , then calculate the value x

Let's determine how much silage and concentrates need to be prepared for 18 cows:

This means that for 18 cows, 180 kg of hay, 216 kg of root crops, 270 kg of silage and 27 kg of concentrates need to be prepared daily.

Problem 3. The housewife makes cherry jam, and puts 2 cups of sugar for 3 cups of cherries. How much sugar should I put in 12 cups of cherries? for 10 glasses of cherries? for a glass of cherries?

Solution

The number of glasses of cherries and the number of glasses of granulated sugar are directly proportional quantities. If the number of glasses of cherries increases several times, the number of glasses of sugar will increase by the same amount.

Let's write down a ratio showing how many times the number of glasses of cherries has increased:

Now let’s write down the ratio showing how many times the number of glasses of sugar has increased:

Let's connect these ratios with an equal sign, get the proportion and find the value x

This means that for 12 cups of cherries you need to put 8 cups of sugar.

Determine the number of cups of sugar for 10 cups of cherries and a cup of cherries

Inverse proportionality problems

To solve problems on inverse proportionality, you can again use a proportion made up of ratios of quantities of the same name.

Unlike direct proportionality, where quantities increase or decrease in the same direction, in inverse proportionality the quantities change inversely to each other.

If one value increases several times, then the other decreases by the same amount. And vice versa, if one value decreases several times, then the other increases by the same amount.

Let's say you need to paint a fence consisting of 8 sheets

One painter will paint all 8 sheets himself

If there are 2 painters, then each will paint 4 sheets.

This is, of course, provided that the painters are honest with each other and fairly divide this work equally between two.

If there are 4 painters, then each will paint 2 sheets

We note that when the number of painters increases several times, the number of sheets per painter decreases by the same amount.

So, we increased the number of painters from 1 to 4. In other words, we quadrupled the number of painters. Let's write this using a relation:

As a result, the number of fence sheets per painter has decreased fourfold. Let's write this using a relation:

Let's connect these relations with an equal sign and get the proportion

“4 painters are to 1 painter as 8 sheets are to 2 sheets”

Problem 2. 15 workers finished finishing the apartments in the new building in 24 days. How many days would it take 18 workers to complete this work?

Solution

The number of workers and the number of days spent on work are inversely proportional. If the number of workers increases several times, the number of days required to complete this work will decrease by the same amount.

Let us write down the ratio of 18 workers to 15 workers. This ratio will show how many times the number of workers has increased

Now let’s write down the second ratio, showing how many times the number of days has decreased. Since the number of days will decrease from 24 days to x days, then the second ratio will be the ratio of the old number of days (24 days) to the new number of days ( x days)

Let's connect the resulting relationships with an equal sign and get the proportion:

From here we find x

This means that 18 workers will complete the necessary work in 20 days.

In general, if you take two inversely proportional quantities and increase one of them by a certain number of times, then the other will decrease by the same amount. Then the ratio of the new value to the old value of the first quantity will be equal to the ratio of the old value to the new value of the second quantity.

So in the previous problem, the old values were 15 working days and 24 days. The number of workers was increased from 15 to 18 (i.e. it was increased several times). As a result, the number of days required to complete the work decreased by the same amount. The new values are 18 working days and 20 days. Then the ratio of the new number of workers to the old number is equal to the ratio of the old number of days to the new number

To create proportions for inverse proportionality problems, you can use the formula:

In relation to our problem, the values of the variables will be as follows:

Where later it became equal to 20.

Problem 2. The speed of the steamboat is related to the speed of the river flow as 36:5. The steamboat moved downstream for 5 hours 10 minutes. How long will it take him to get back?

Solution

The ship's own speed is 36 km/h. The river flow speed is 5 km/h. Since the steamer was moving with the current of the hand, its speed was 36 + 5 = 41 km/h. Travel time was 5 hours 10 minutes. For convenience, we express the time in minutes:

5 hours 10 minutes = 300 minutes + 10 minutes = 310 minutes

Since on the way back the ship was moving against the flow of the river, its speed was 36 − 5 = 31 km/h.

The speed of the ship and the time of its movement are inversely proportional quantities. If the speed decreases several times, the time of its movement will increase by the same amount.

Let's write down the ratio showing how many times the speed of movement has decreased:

Now let’s write down the second ratio, showing how many times the movement time has increased. Since the new time x will be greater than the old time, we will write the time in the numerator of the ratio x, and the denominator is the old time equal to three hundred and ten minutes

Let's connect the resulting ratios with an equal sign and get the proportion. From here we find the value x

410 minutes is 6 hours and 50 minutes. This means that the ship will take 6 hours and 50 minutes to return.

Problem 3. There were 15 people working on the road repair, and they had to finish the job in 12 days. On the fifth day, several more workers arrived in the morning, and the remaining work was completed in 6 days. How many additional workers arrived?

Solution

Subtract 4 days worked from 12 days. This way we will determine how many more days the fifteen workers have left to work

12 days − 4 days = 8 days

On the fifth day additional arrivals x workers. Then the total number of workers became 15+ x .

The number of workers and the number of days required to complete the work are inversely proportional. If the number of workers increases several times, the number of days will decrease by the same amount.

Let's write down a ratio showing how many times the number of workers has increased:

Now let’s write down how many times the number of days required to complete the work has decreased:

Let's connect these relations with an equal sign and get proportion. From here you can calculate the value x

This means that 5 additional workers arrived.

Scale

Scale is the ratio of the length of a segment in the image to the length of the corresponding segment on the ground.

Let's assume that the distance from home to school is 8 km. Let's try to draw a plan of the area, where the house, school and the distance between them will be indicated. But we cannot depict on paper a distance of 8 km, since it is quite large. But we can reduce this distance several times so that it fits on paper.

Let kilometers on the ground on our plan be expressed in centimeters. Let's convert 8 kilometers to centimeters, we get 800,000 centimeters.

Let’s reduce 800,000 cm by a hundred thousand times:

800,000 cm: 100,000 cm = 8 cm

8 cm is the distance from home to school, reduced by a hundred thousand times. Now you can easily draw a house and a school on paper, the distance between them will be 8 cm.

These 8 cm refer to the real 800,000 cm. So we write it using the ratio:

8: 800 000

One of the properties of a relation states that the relation does not change if its members are multiplied or divided by the same number.

In order to simplify the ratio 8: 800,000, both of its terms can be divided by 8. Then we get the ratio 1: 100,000. We call this ratio the scale. This ratio shows that one centimeter on the plan relates (or corresponds) to one hundred thousand centimeters on the ground.

Therefore, in our drawing it is necessary to indicate that the plan is drawn up on a scale of 1: 100,000

1 cm on the plan refers to 100,000 cm on the ground;
2 cm on the plan refers to 200,000 cm on the ground;
3 cm on the plan refers to 300,000 on the ground, etc.

For any map or plan it is indicated at what scale they were made. This scale allows you to determine the actual distance between objects.

So, our plan is drawn up on a scale of 1: 100,000. On this plan, the distance between home and school is 8 cm. To calculate the real distance between home and school, you need to increase 8 cm by 100,000 times. In other words, multiply 8 cm by 100,000

8 cm × 100,000 = 800,000 cm

We get 800,000 cm or 8 km, if we convert centimeters to kilometers.

Let's say that there is a tree between the house and the school. On the plan, the distance between the school and this tree is 4 cm.

Then the actual distance between the house and the tree will be 4 cm × 100,000 = 400,000 cm or 4 km.

Distance on the ground can be determined using proportion. In our example, the distance between home and school will be calculated using the following proportion:

1 cm on the plan is related to 100,000 cm on the ground, just as 8 cm on the plan is related to x cm on the ground.

From this proportion we find out that the value x equals 800000 cm.

Example 2. On the map, the distance between the two cities is 8.5 cm. Determine the real distance between the cities if the map is drawn up on a scale of 1: 1,000,000.

Solution

A scale of 1:1,000,000 indicates that 1 cm on the map corresponds to 1,000,000 cm on the ground. Then 8.5 cm will correspond x cm on the ground. Let's make the proportion 1 to 1000000 as 8.5 to x

1 km contains 100,000 cm. Then 8,500,000 cm will contain

Or you can think like this. The distance on the map and the distance on the ground are directly proportional quantities. If the distance on the map increases several times, the distance on the ground will increase by the same amount. Then the proportion will take the following form. The first ratio will show how many times the distance on the ground is greater than the distance on the map:

The second ratio will show that the distance on the ground is the same number of times greater than 8.5 cm on the map:

From here x equal to 8,500,000 cm or 85 km.

Problem 3. The length of the Neva River is 74 km. What is its length on a map whose scale is 1: 2,000,000

Solution

A scale of 1: 2,000,000 means that 1 cm on the map corresponds to 2,000,000 cm on the ground.

And 74 km is 74 × 100,000 = 7,400,000 cm on the ground. By reducing 7,400,000 to 2,000,000, we will determine the length of the Neva River on the map

7,400,000: 2,000,000 = 3.7 cm

This means that on a map whose scale is 1: 2,000,000, the length of the Neva River is 3.7 cm.

Let's write the solution using a proportion. The first ratio will show how many times the length on the map is less than the length on the ground:

The second ratio will show that 74 km (7,400,000 cm) decreased by the same amount:

From here we find x equal to 3.7 cm

Problems to solve independently

Problem 1. From 21 kg of cottonseed, 5.1 kg of oil was obtained. How much oil will be obtained from 7 kg of cottonseed?

Solution

Let x kg of oil can be obtained from 7 kg of cottonseed. The mass of cotton seed and the mass of the resulting oil are directly proportional quantities. Then reducing the cotton seed from 21 kg to 7 kg will lead to a decrease in the resulting oil by the same amount.

Answer: 7 kg of cottonseed will yield 1.7 kg of oil.

Problem 2. On a certain section of the railway track, old rails 8 m long were replaced with new ones 12 m long. How many new twelve-meter rails will be required if 360 old rails were removed?

Solution

The length of the section where the rails are being replaced is 8 × 360 = 2880 m.

Let x twelve-meter rails are required for replacement. Increasing the length of one rail from 8 m to 12 m will lead to a reduction in the number of rails from 360 to x things. In other words, the length of the rail and their number are inversely proportional

Answer: replacing old rails will require 240 new ones.

Task 3. 60% of the students in the class went to the cinema, and the remaining 12 people went to the exhibition. How many students are in the class?

Solution

If 60% of the students went to the cinema, and the remaining 12 people went to the exhibition, then 40% of the students will account for 12 people who went to the exhibition. Then you can create a proportion in which 12 students treat 40% the same way as everyone else x students are 100%

Or you can create a proportion consisting of ratios of quantities of the same name. Enrollment numbers and percentages vary in direct proportion. Then we can write that how many times the number of participants increased, how many times did the percentage increase

Problem 5. The pedestrian spent 2.5 hours on the journey, moving at a speed of 3.6 km/h. How much time will a pedestrian spend on the same path if his speed is 4.5 km/h

Solution

Speed and time are inversely proportional quantities. When the speed increases several times, the movement time will decrease by the same amount.

Let's write down a ratio showing how many times the pedestrian's speed has increased:

Let's write down a ratio showing that the time of movement has decreased by the same amount:

Let's connect these ratios with an equal sign, get the proportion and find the value x

Or you can use the ratios of quantities of the same name. The number of machines produced and the percentage of these machines accounted for are directly proportional. When the number of machines increases several times, the percentage increases by the same amount. Then we can write that 230 machines are so many times more than x machines, how many times more is 115% than 100%

Answer: According to the plan, the plant was supposed to produce 200 machines.

Did you like the lesson?
Join our new VKontakte group and start receiving notifications about new lessons

Proportion – equality of two relations, i.e. equality of the form a: b = c: d , or, in other notations, equality

If a : b = c : d, That a And d called extreme, A b And c - averagemembers proportions.

There is no escape from “proportion”; many tasks cannot be done without it. There is only one way out - to deal with this relationship and use proportion as a lifesaver.

Before we begin to consider proportion problems, it is important to remember the basic rule of proportion:

In proportion

the product of the extreme terms is equal to the product of the middle terms

If some quantity in a proportion is unknown, it will be easy to find it based on this rule.

For example,

That is, the unknown value of the proportion - the value of the fraction, in the denominator which is the number that stands opposite the unknown quantity , in the numerator – the product of the remaining terms of the proportion (regardless of where this unknown quantity stands).

Task 1.

From 21 kg of cottonseed, 5.1 kg of oil was obtained. How much oil will be obtained from 7 kg of cottonseed?

Solution:

We understand that a decrease in the weight of the seed by a certain factor entails a decrease in the weight of the resulting oil by the same amount. That is, the quantities are directly related.

Let's fill out the table:

An unknown quantity is the value of a fraction, in the denominator of which - 21 - the value opposite the unknown in the table, in the numerator - the product of the remaining members of the proportion table.

Therefore, we find that 1.7 kg of oil will come out of 7 kg of seed.

To Right When filling out the table, it is important to remember the rule:

Identical names must be written below each other. We write percentages under percentages, kilograms under kilograms, etc.

Task 2.

Convert to radians.

Solution:

We know that . Let's fill out the table:

Answer:

Task 3.

A circle is depicted on checkered paper. What is the area of the circle if the area of the shaded sector is 27?

Solution:

It is clearly seen that the unshaded sector corresponds to the angle in (for example, because the sides of the sector are formed by the bisectors of two adjacent right angles). And since the entire circle is , then the shaded sector accounts for .

Let's make a table:

Where does the area of a circle come from?

Answer:

Task 4.After 82% of the entire field had been plowed, there was still 9 hectares left to plow. What is the area of the entire field?

Solution:

The entire field is 100%, and since 82% is plowed, then 100%-82%=18% of the field remains to be plowed.

Fill out the table:

From where we get that the entire field is (ha).

Answer:

And the next task is an ambush.

Task 5.

A passenger train covered the distance between two cities at a speed of 80 km/h in 3 hours. How many hours will it take a freight train to cover the same distance at a speed of 60? km/h?

Solution:

If you solve this problem similarly to the previous one, you will get the following:

the time it takes for a freight train to travel the same distance as a passenger train is hours. That is, it turns out that walking at a lower speed, he covers (in the same time) the distance faster than a train with a higher speed.

What is the error in reasoning?

So far we have considered problems where the quantities were directly proportional to each other , that is height of the same value several times, gives height the second quantity associated with it by the same amount (similarly with a decrease, of course). And here we have a different situation: the speed of a passenger train more the speed of a freight train is several times higher, but the time required to cover the same distance is required by a passenger train smaller as many times as a freight train. That is, values to each other inversely proportional .

The scheme that we have used so far needs to be slightly changed in this case.

Solution:

We reason like this:

A passenger train traveled for 3 hours at a speed of 80 km/h, therefore it traveled km. This means that a freight train will cover the same distance in an hour.

That is, if we were making a proportion, we should have swapped the cells of the right column first. Would get: h.

Answer: .

That's why, please be careful when drawing up the proportions. First, figure out what kind of dependence you are dealing with - direct or inverse.

A proportion is a mathematical expression that compares two or more numbers to each other. Proportions can compare absolute values and quantities or parts of a larger whole. Proportions can be written and calculated in several different ways, but the basic principle is the same.

Steps

Part 1

What is proportion

Find out what proportions are for. Proportions are used both in scientific research and in everyday life to compare different quantities and quantities. In the simplest case, two numbers are compared, but a proportion can include any number of quantities. When comparing two or more quantities, you can always use proportion. Knowing how quantities relate to each other allows, for example, to write down chemical formulas or recipes for various dishes. Proportions will be useful to you for a variety of purposes.

Learn what proportion means. As noted above, proportions allow us to determine the relationship between two or more quantities. For example, if you need 2 cups of flour and 1 cup of sugar to make cookies, we say that there is a 2 to 1 ratio between the amount of flour and sugar.
- Proportions can be used to show how different quantities relate to each other, even if they are not directly related (unlike a recipe). For example, if there are five girls and ten boys in a class, the ratio of girls to boys is 5 to 10. In this case, one number is not dependent on or directly related to the other: the proportion may change if someone leaves the class or vice versa , new students will come to it. A proportion simply allows you to compare two quantities.
Notice the different ways of expressing proportions. Proportions can be written in words or using mathematical symbols.
- In everyday life, proportions are more often expressed in words (as above). Proportions are used in a variety of fields, and unless your profession is related to mathematics or other science, this is the most common way you will come across this way of writing proportions.
- Proportions are often written using a colon. When comparing two numbers using a proportion, they can be written with a colon, for example 7:13. If more than two numbers are being compared, a colon is placed consecutively between each two numbers, for example 10:2:23. In the above example for a class, we are comparing the number of girls and boys, with 5 girls: 10 boys. Thus, in this case the proportion can be written as 5:10.
- Sometimes a fraction sign is used when writing proportions. In our class example, the ratio of 5 girls to 10 boys would be written as 5/10. In this case, you should not read the “divide” sign and you must remember that this is not a fraction, but a ratio of two different numbers.
Part 2
Operations with proportions
1. Reduce the proportion to its simplest form. Proportions can be simplified, like fractions, by reducing their members by a common divisor. To simplify a proportion, divide all numbers included in it by common divisors. However, we should not forget about the initial values that led to this proportion.
  - In the example above with a class of 5 girls and 10 boys (5:10), both sides of the proportion have a common factor of 5. Dividing both quantities by 5 (the greatest common factor) gives a ratio of 1 girl to 2 boys (i.e. 1:2) . However, when using a simplified proportion, you should remember the original numbers: there are not 3 students in the class, but 15. The reduced proportion only shows the ratio between the number of girls and boys. For every girl there are two boys, but this does not mean that there is 1 girl and 2 boys in the class.
  - Some proportions cannot be simplified. For example, the ratio 3:56 cannot be reduced, since the quantities included in the proportion do not have a common divisor: 3 is a prime number, and 56 is not divisible by 3.
2. To “scale” proportions can be multiplied or divided. Proportions are often used to increase or decrease numbers in proportion to each other. Multiplying or dividing all quantities included in a proportion by the same number keeps the relationship between them unchanged. Thus, the proportions can be multiplied or divided by the “scale” factor.
  - Let's say a baker needs to triple the amount of cookies he bakes. If flour and sugar are taken in a ratio of 2 to 1 (2:1), to triple the amount of cookies, this proportion should be multiplied by 3. The result will be 6 cups of flour to 3 cups of sugar (6:3).
  - You can do the opposite. If the baker needs to reduce the amount of cookies by half, both parts of the proportion should be divided by 2 (or multiplied by 1/2). The result is 1 cup of flour per half cup (1/2, or 0.5 cup) of sugar.
3. Learn to find an unknown quantity using two equivalent proportions. Another common problem for which proportions are widely used is finding an unknown quantity in one of the proportions if a second proportion similar to it is given. The rule for multiplying fractions greatly simplifies this task. Write each proportion as a fraction, then equate these fractions to each other and find the required quantity.
  - Let's say we have a small group of students consisting of 2 boys and 5 girls. If we want to maintain the ratio between boys and girls, how many boys should there be in a class of 20 girls? First, let's create both proportions, one of which contains the unknown quantity: 2 boys: 5 girls = x boys: 20 girls. If we write the proportions as fractions, we get 2/5 and x/20. After multiplying both sides of the equality by the denominators, we obtain the equation 5x=40; divide 40 by 5 and ultimately find x=8.
Part 3
Troubleshooting
1. When operating with proportions, avoid addition and subtraction. Many problems with proportions sound like the following: “To prepare a dish you need 4 potatoes and 5 carrots. If you want to use 8 potatoes, how many carrots will you need?” Many people make the mistake of trying to simply add up the corresponding values. However, to maintain the same proportion, you should multiply rather than add. Here is the wrong and correct solution to this problem:
  - Incorrect method: “8 - 4 = 4, that is, 4 potatoes were added to the recipe. This means that you need to take the previous 5 carrots and add 4 to them so that... something is wrong! Proportions work differently. Let's try again".
  - Correct method: “8/4 = 2, that is, the number of potatoes has doubled. This means that the number of carrots should be multiplied by 2. 5 x 2 = 10, that is, 10 carrots must be used in the new recipe.”
2. Convert all values to the same units. Sometimes the problem occurs because quantities have different units. Before writing down the proportion, convert all quantities into the same units. For example:
  - The dragon has 500 grams of gold and 10 kilograms of silver. What is the ratio of gold to silver in dragon hoards?
  - Grams and kilograms are different units of measurement, so they should be unified. 1 kilogram = 1,000 grams, that is, 10 kilograms = 10 kilograms x 1,000 grams/1 kilogram = 10 x 1,000 grams = 10,000 grams.
  - So the dragon has 500 grams of gold and 10,000 grams of silver.
  - The ratio of the mass of gold to the mass of silver is 500 grams of gold/10,000 grams of silver = 5/100 = 1/20.
3. Write down the units of measurement in the solution to the problem. In problems with proportions, it is much easier to find an error if you write down its units of measurement after each value. Remember that if the numerator and denominator have the same units of measurement, they cancel. After all possible abbreviations, your answer should have the correct units of measurement.
  - For example: given 6 boxes, and in every three boxes there are 9 balls; how many balls are there in total?
  - Incorrect method: 6 boxes x 3 boxes/9 marbles = ... Hmm, nothing is reduced, and the answer comes out to be “boxes x boxes / marbles“. It does not make sense.
  - Correct method: 6 boxes x 9 balls/3 boxes = 6 boxes x 3 balls/1 box = 6 x 3 balls/1= 18 balls.

Problem 1. The thickness of 300 sheets of printer paper is 3.3 cm. What thickness will a pack of 500 sheets of the same paper have?

Solution. Let x cm be the thickness of a stack of paper of 500 sheets. There are two ways to find the thickness of one sheet of paper:

3,3: 300 or x : 500.

Since the sheets of paper are the same, these two ratios are equal. We get the proportion ( reminder: proportion is the equality of two ratios):

x=(3.3 · 500): 300;

x=5.5. Answer: pack 500 sheets of paper have a thickness 5.5 cm.

This is a classic reasoning and design of a solution to a problem. Such problems are often included in test tasks for graduates, who usually write the solution in the following form:

or they decide orally, reasoning like this: if 300 sheets have a thickness of 3.3 cm, then 100 sheets have a thickness 3 times less. Divide 3.3 by 3, we get 1.1 cm. This is the thickness of a 100-sheet pack of paper. Therefore, 500 sheets will have a thickness 5 times greater, therefore, we multiply 1.1 cm by 5 and get the answer: 5.5 cm.

Of course, this is justified, since the time for testing graduates and applicants is limited. However, in this lesson we will reason and write down the solution as it should be done in 6 class.

Task 2. How much water is contained in 5 kg of watermelon, if it is known that watermelon consists of 98% water?

Solution.

The entire mass of the watermelon (5 kg) is 100%. Water will be x kg or 98%. There are two ways to find how many kg are in 1% of the mass.

5: 100 or x : 98. We get the proportion:

5: 100 = x : 98.

x=(5 · 98): 100;

x=4.9 Answer: 5kg watermelon contains 4.9 kg water.

The mass of 21 liters of oil is 16.8 kg. What is the mass of 35 liters of oil?

Solution.

Let the mass of 35 liters of oil be x kg. Then you can find the mass of 1 liter of oil in two ways:

16,8: 21 or x : 35. We get the proportion:

16,8: 21=x : 35.

Find the middle term of the proportion. To do this, we multiply the extreme terms of the proportion ( 16,8 And 35 ) and divide by the known average term ( 21 ). Let's reduce the fraction by 7 .

Multiply the numerator and denominator of the fraction by 10 so that the numerator and denominator contain only natural numbers. We reduce the fraction by 5 (5 and 10) and on 3 (168 and 3).